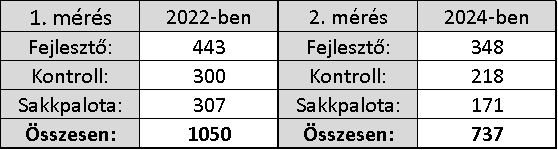
Students from the psychology program at ELTE are examining the measurement data obtained using the Sensorimotor and Cognitive Profile Test applied in our research project. Two theses have been completed on this topic:
- Evelin Haász: The relationship between cognitive and sensorimotor abilities and school performance among first-grade students – the thesis can be read here.
- Hanga Kiss: The relationship between working memory, fluid intelligence, and phonemic awareness and first-grade school performance – the thesis can be read here.